This specification is presented in a two column format.
The subject content is split into three sections for each of the subject areas: biology, chemistry and physics.
The left hand column contains the subject content that all students must cover, and that can be assessed in the written papers.
The right hand column exemplifies some of the key opportunities for the following skills to be developed throughout the course: WS refers to Working scientifically , MS refers to Mathematical requirements and AT refers to Use of apparatus and techniques . These are not the only opportunities. Teachers are encouraged to introduce all of these skills where appropriate throughout the course.
Each topic begins with an overview. The overview puts the topic into a broader context and is intended to encourage an overarching approach to both the teaching and learning of each of the topic areas. It is not directly assessed. Any assessable content in this overview is replicated in the left hand column.
It is good practice to teach and develop all of the mathematical skills throughout the course. Some mathematical skills will only be assessed in certain subject areas. These are detailed in Mathematical requirements .
Science is a practical subject. Details of the assessment of required practicals can be found in Required practical activities .
Working scientifically and Use of apparatus and techniques skills will be assessed across all papers.
Content that is only applicable to Higher Tier is indicated by (HT only) either next to the topic heading where it applies to the whole topic or immediately preceding each paragraph or bullet point as applicable.
Questions in paper 2 may draw on an understanding of energy changes and transfers due to heating, mechanical and electrical work and the concept of energy conservation from sections Energy and Electricity .
6.1 Energy
The concept of energy emerged in the 19th century. The idea was used to explain the work output of steam engines and then generalised to understand other heat engines. It also became a key tool for understanding chemical reactions and biological systems.
Limits to the use of fossil fuels and global warming are critical problems for this century. Physicists and engineers are working hard to identify ways to reduce our energy usage.
6.1.1 Energy changes in a system, and the ways energy is stored before and after such changes
6.1.1.1 Energy stores and systems
Content | Key opportunities for skills development |
|---|
A system is an object or group of objects. There are changes in the way energy is stored when a system changes. Students should be able to describe all the changes involved in the way energy is stored when a system changes, for common situations. F or example: - an object projected upwards
- a moving object hitting an obstacle
- an object accelerated by a constant force
- a vehicle slowing down
- bringing water to a boil in an electric kettle.
Throughout this section on Energy students should be able to calculate the changes in energy involved when a system is changed by: - heating
- work done by forces
- work done when a current flows
| The link between work done (energy transfer) and current flow in a circuit is covered in Work done and energy transfer . WS 4.5 |
- use calculations to show on a common scale how the overall energy in a system is redistributed when the system is changed.
| WS 1.2 , 4.3, 4.5, 4.6 MS 1a, c, 3b, c |
6.1.1.2 Changes in energy
Content | Key opportunities for skills development |
|---|
Students should be able to calculate the amount of energy associated with a moving object, a stretched spring and an object raised above ground level. | WS 1.2, 4.3, 4.4, 4.6 MS 1a, c, 3b, c |
The kinetic energy of a moving object can be calculated using the equation: kinetic energy, E k , in joules, J mass, m , in kilograms, kg speed, v , in metres per second, m/s The amount of elastic potential energy stored in a stretched spring can be calculated using the equation: | MS 3b, c Students should be able to recall and apply this equation. |
(assuming the limit of proportionality has not been exceeded) elastic potential energy, E e , in joules, J spring constant, k , in newtons per metre, N/m extension, e , in metres, m The amount of gravitational potential energy gained by an object raised above ground level can be calculated using the equation: | MS 3b, c Students should be able to apply this equation which is given on the Physics equation sheet . |
gravitational potential energy, E p , in joules, J mass, m , in kilograms, kg gravitational field strength, g , in newtons per kilogram, N/kg (In any calculation the value of the gravitational field strength ( g ) will be given). height, h , in metres, m | MS 3b, c Students should be able to recall and apply this equation. AT 1 Investigate the transfer of energy from a gravitational potential energy store to a kinetic energy store. |
6.1.1.3 Energy changes in systems
Content | Key opportunities for skills development |
|---|
The amount of energy stored in or released from a system as its temperature changes can be calculated using the equation: | |
change in thermal energy, ∆ E , in joules, J mass, m , in kilograms, kg specific heat capacity, c , in joules per kilogram per degree Celsius, J/kg °C temperature change, ∆ θ , in degrees Celsius, °C The specific heat capacity of a substance is the amount of energy required to raise the temperature of one kilogram of the substance by one degree Celsius. | MS 3b, c Students should be able to apply this equation which is given on the Physics equation sheet . This equation and specific heat capacity are also included in Temperature changes in a system and specific heat capacity . |
Required practical activity 14 : an investigat ion to determine the specific heat capacity of one or more materials. The investigation will involve linking the decrease of one energy store (or work done) to the increase in temperature and subsequent increase in thermal energy stored.
AT skills covered by this practical activity: physics AT 1 and 5.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.1.1.4 Power
Content | Key opportunities for skills development |
|---|
Power is defined as the rate at which energy is transferred or the rate at which work is done. power, P , in watts, W energy transferred, E , in joules, J time, t , in seconds, s work done, W , in joules, J An energy transfer of 1 joule per second is equal to a power of 1 watt. Students should be able to give examples that illustrate the definition of power eg comparing two electric motors that both lift the same weight through the same height but one does it faster than the other. | MS 3b, c Students should be able to recall and apply both equations. |
6.1.2 Conservation and dissipation of energy
6.1.2.1 Energy transfers in a system
Content | Key opportunities for skills development |
|---|
Energy can be transferred usefully, stored or dissipated, but cannot be created or destroyed. Students should be able to describe with examples where there are energy transfers in a closed system, that there is no net change to the total energy. Students should be able to describe, with examples, how in all system changes energy is dissipated, so that it is stored in less useful ways. This energy is often described as being ‘wasted’. | |
Students should be able to explain ways of reducing unwanted energy transfers, for example through lubrication and the use of thermal insulation. The higher the thermal conductivity of a material the higher the rate of energy transfer by conduction across the material. Students should be able to describe how the rate of cooling of a building is affected by the thickness and thermal conductivity of its walls. Students do not need to know the definition of thermal conductivity. | WS 1.4 AT 1, 5 Investigate thermal conductivity using rods of different materials. |
6.1.2.2 Efficiency
Content | Key opportunities for skills development |
|---|
The energy efficiency for any energy transfer can be calculated using the equation: Efficiency may also be calculated using the equation: | MS 3b, c Students should be able to recall and apply both equations. MS 1a, c, 3b, c Students may be required to calculate or use efficiency values as a decimal or as a percentage. |
(HT only) Students should be able to describe ways to increase the efficiency of an intended energy transfer. | (HT only) WS 1.4 |
6.1.3 National and global energy resources
Content | Key opportunities for skills development |
|---|
The main energy resources available for use on Earth include: fossil fuels (coal, oil and gas), nuclear fuel, bio-fuel, wind, hydro-electricity, geothermal, the tides, the Sun and water waves. A renewable energy resource is one that is being (or can be) replenished as it is used. The uses of energy resources include: transport, electricity generation and heating. Students should be able to: - describe the main energy sources available
- distinguish between energy resources that are renewable and energy resources that are non-renewable
- compare ways that different energy resources are used, the uses to include transport, electricity generation and heating
- understand why some energy resources are more reliable than others
| WS 4.4 |
- describe the environmental impact arising from the use of different energy resources
| WS 1.3, 1.4 |
- explain patterns and trends in the use of energy resources.
| WS 3.5 |
Descriptions of how energy resources are used to generate electricity are not required. | |
Students should be able to: - consider the environmental issues that may arise from the use of different energy resources
- show that science has the ability to identify environmental issues arising from the use of energy resources but not always the power to deal with the issues because of political, social, ethical or economic considerations.
| WS 1.3, 1.4, 4.4 MS 1c, 2c, 4a |
6.2 Electricity
Electric charge is a fundamental property of matter everywhere. Understanding the difference in the microstructure of conductors, semiconductors and insulators makes it possible to design components and build electric circuits. Many circuits are powered with mains electricity, but portable electrical devices must use batteries of some kind.
Electrical power fills the modern world with artificial light and sound, information and entertainment, remote sensing and control. The fundamentals of electromagnetism were worked out by scientists of the 19th century. However, power stations, like all machines, have a limited lifetime. If we all continue to demand more electricity this means building new power stations in every generation – but what mix of power stations can promise a sustainable future?
6.2.1 Current, potential difference and resistance
6.2.1.1 Standard circuit diagram symbols
Content | Key opportunities for skills development |
|---|
Circuit diagrams use standard symbols. 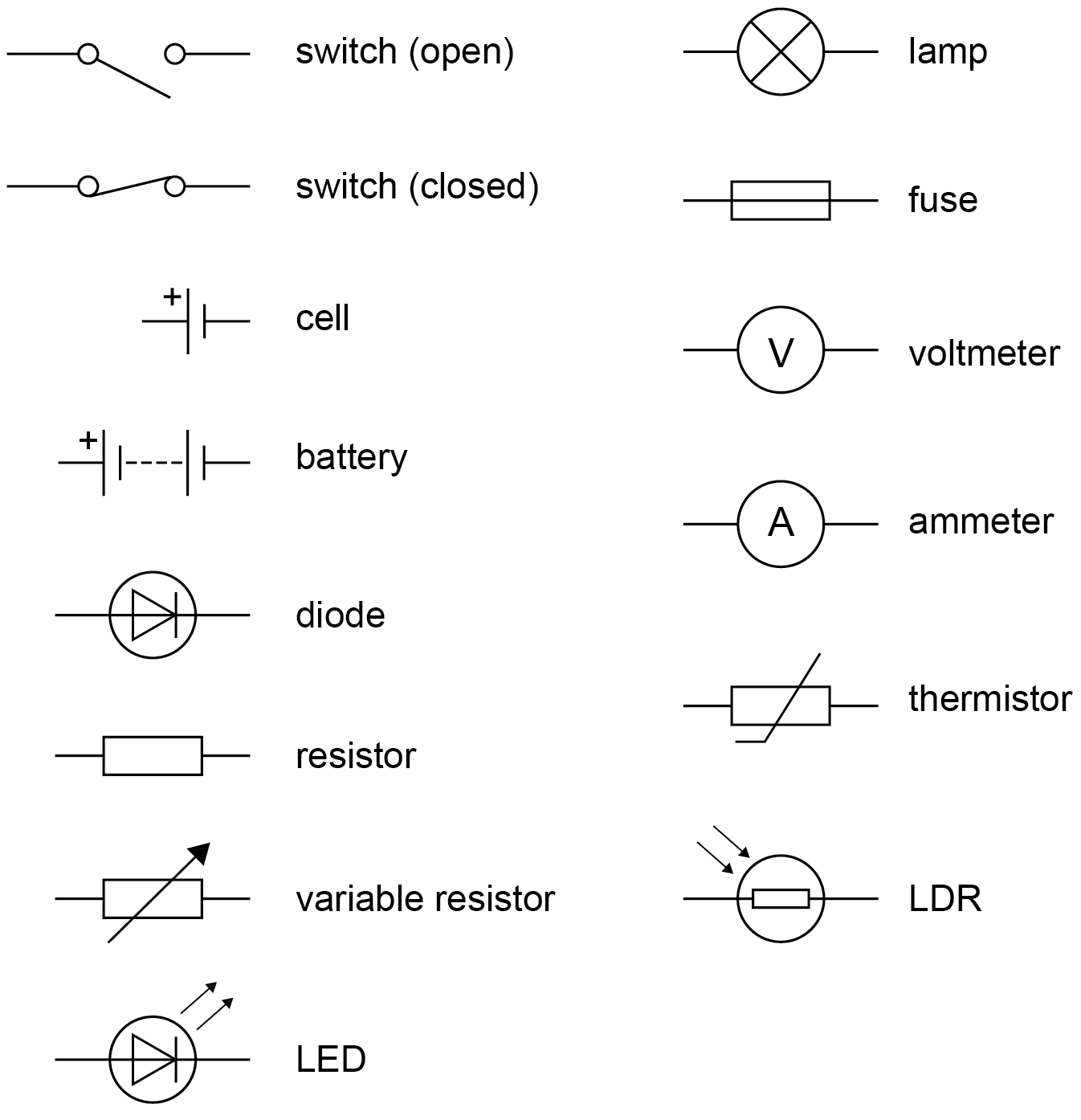 Students should be able to draw and interpret circuit diagrams . | WS 1.2 |
6.2.1.2 Electrical charge and current
Content | Key opportunities for skills development |
|---|
For electrical charge to flow through a closed circuit the circuit must include a source of potential difference. | |
Electric current is a flow of electrical charge. The size of the electric current is the rate of flow of electrical charge. Charge flow, current and time are linked by the equation: charge flow, Q , in coulombs, C current, I , in amperes, A (amp is acceptable for ampere) time, t , in seconds, s A current has the same value at any point in a single closed loop . | MS 3b, c Students should be able to recall and apply this equation. |
6.2.1.3 Current, resistance and potential difference
Content | Key opportunities for skills development |
|---|
The current ( I ) through a component depends on both the resistance ( R ) of the component and the potential difference ( V ) across the component. The greater the resistance of the component the smaller the current for a given potential difference (pd) across the component. Questions will be set using the term potential difference. Students will gain credit for the correct use of either potential difference or voltage. | |
Current, potential difference or resistance can be calculated using the equation: potential difference, V , in volts, V current, I , in amperes, A (amp is acceptable for ampere) resistance, R , in ohms, Ω | MS 3b, c Students should be able to recall and apply this equation. |
- the length of a wire at constant temperature
- combinations of resistors in series and parallel.
AT skills covered by this practical activity: physics AT 1, 6 and 7.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.2.1.4 Resistors
Content | Key opportunities for skills development |
|---|
Students should be able to explain that, for some resistors, the value of R remains constant but that in others it can change as the current changes. The current through an ohmic conductor (at a constant temperature) is directly proportional to the potential difference across the resistor. This means that the resistance remains constant as the current changes. 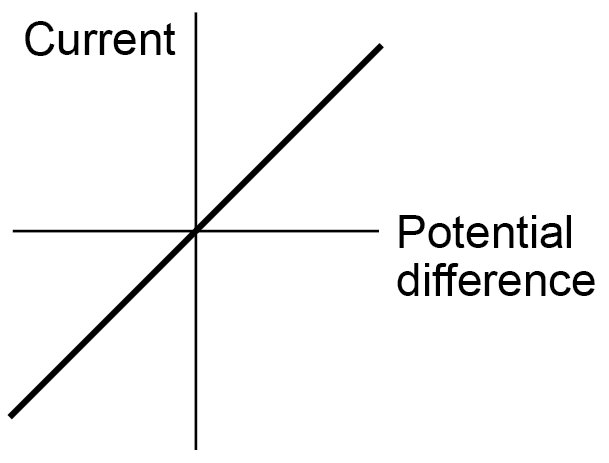 The resistance of components such as lamps, diodes, thermistors and LDRs is not constant; it changes with the current through the component. The resistance of a filament lamp increases as the temperature of the filament increases.  The current through a diode flows in one direction only. The diode has a very high resistance in the reverse direction. 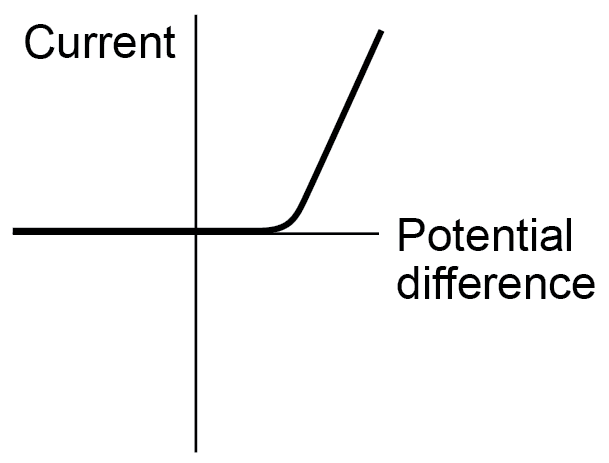 | |
The resistance of a thermistor decreases as the temperature increases. The applications of thermistors in circuits eg a thermostat is required. The resistance of an LDR decreases as light intensity increases. | |
The application of LDRs in circuits eg switching lights on when it gets dark is required. Students should be able to: | WS 1.2, 1.4 |
- explain the design and use of a circuit to measure the resistance of a component by measuring the current through, and potential difference across, the component
- draw an appropriate circuit diagram using correct circuit symbols.
| AT 6 Investigate the relationship between the resistance of a thermistor and temperature. Investigate the relationship between the resistance of an LDR and light intensity. |
Students should be able to use graphs to explore whether circuit elements are linear or non-linear and relate the curves produced to the ir function and properties. | WS 1.2, 1.4 MS 4c, 4d, 4e |
Required practical activity 16: use circuit diagrams to construct appropriate circuits to investigate the I–V characteristics of a variety of circuit elements, including a filament lamp, a diode and a resistor at constant temperature.
AT skills covered by this practical activity: physics AT 6 and 7.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.2.2 Series and parallel circuits
Content | Key opportunities for skills development |
|---|
There are two ways of joining electrical components, in series and in parallel. Some circuits include both series and parallel parts. For components connected in series: - there is the same current through each component
- the total potential difference of the power supply is shared between the components
- the total resistance of two components is the sum of the resistance of each component.
| |
resistance, R , in ohms, Ω For components connected in parallel: - the potential difference across each component is the same
- the total current through the whole circuit is the sum of the currents through the separate components
- the total resistance of two resistors is less than the resistance of the smallest individual resistor.
Students should be able to: | MS 1c, 3b, 3c, 3d |
- use circuit diagrams to construct and check series and parallel circuits that include a variety of common circuit components
- describe the difference between series and parallel circuits
- explain qualitatively why adding resistors in series increases the total resistance whilst adding resistors in parallel decreases the total resistance
| AT 7 |
- explain the design and use of dc series circuits for measurement and testing purposes
| WS 1.4 |
- calculate the currents, potential differences and resistances in dc series circuits
- solve problems for circuits which include resistors in series using the concept of equivalent resistance.
Students are not required to calculate the total resistance of two resistors joined in parallel. | MS 1c, 3b, c, d |
6.2.3 Domestic uses and safety
6.2.3.1 Direct and alternating potential difference
Content | Key opportunities for skills development |
|---|
Mains electricity is an ac supply. In the United Kingdom the domestic electricity supply has a frequency of 50 Hz and is about 230 V. Students should be able to explain the difference between direct and alternating potential difference. | |
6.2.3.2 Mains electricity
Content | Key opportunities for skills development |
|---|
Most electrical appliances are connected to the mains using three-core cable. The insulation covering each wire is colour coded for easy identification: live wire – brown neutral wire – blue earth wire – green and yellow stripes. The live wire carries the alternating potential difference from the supply. The neutral wire completes the circuit. The earth wire is a safety wire to stop the appliance becoming live. The potential difference between the live wire and earth (0 V) is about 230 V. The neutral wire is at, or close to, earth potential (0 V). The earth wire is at 0 V, it only carries a current if there is a fault. Students should be able to explain: - that a live wire may be dangerous even when a switch in the mains circuit is open
- the dangers of providing any connection between the live wire and earth.
| WS 1.5 |
6.2.4 Energy transfers
6.2.4.1 Power
Content | Key opportunities for skills development |
|---|
Students should be able to explain how the power transfer in any circuit device is related to the potential difference across it and the current through it, and to the energy changes over time : power, P , in watts, W potential difference, V , in volts, V current, I , in amperes, A (amp is acceptable for ampere) resistance, R , in ohms, Ω | MS 3b, c WS 4.5 Students should be able to recall and apply both equations. |
6.2.4.2 Energy transfers in everyday appliances
Content | Key opportunities for skills development |
|---|
Everyday electrical appliances are designed to bring about energy transfers. The amount of energy an appliance transfers depends on how long the appliance is switched on for and the power of the appliance. Students should be able to describe how different domestic appliances transfer energy from batteries or ac mains to the kinetic energy of electric motors or the energy of heating devices. Work is done when charge flows in a circuit. The amount of energy transferred by electrical work can be calculated using the equation: | |
energy transferred, E , in joules, J power, P , in watts, W time, t , in seconds, s charge flow, Q , in coulombs, C potential difference, V , in volts, V | MS 3b, c Students should be able to recall and apply both equations. WS 1.4 |
Students should be able to explain how the power of a circuit device is related to: - the potential difference across it and the current through it
- the energy transferred over a given time.
Students should be able to describe, with examples, the relationship between the power ratings for domestic electrical appliances and the changes in stored energy when they are in use. | WS 1.2 |
6.2.4.3 The National Grid
Content | Key opportunities for skills development |
|---|
The National Grid is a system of cables and transformers linking power stations to consumers. | |
Electrical power is transferred from power stations to consumers using the National Grid. Step-up transformers are used to increase the potential difference from the power station to the transmission cables then step-down transformers are used to decrease, to a much lower value, the potential difference for domestic use. Students should be able to explain why the National Grid system is an efficient way to transfer energy. Higher tier only: Students should be able to select and use the equation: potential difference across primary coil x current in primary coil = potential difference across secondary coil x current in secondary coil as given on the equation sheet. | WS 1.4 Detailed knowledge of the structure of a transformer is not required. |
6.3 Particle model of matter
The particle model is widely used to predict the behaviour of solids, liquids and gases and this has many applications in everyday life. It helps us to explain a wide range of observations and engineers use these principles when designing vessels to withstand high pressures and temperatures, such as submarines and spacecraft. It also explains why it is difficult to make a good cup of tea high up a mountain!
6.3.1 Changes of state and the particle model
6.3.1.1 Density of materials
Content | Key opportunities for skills development |
|---|
The density of a material is defined by the equation: density, ρ , in kilograms per metre cubed, kg/m3 mass, m , in kilograms, kg volume, V , in metres cubed, m3 The particle model can be used to explain - the different states of matter
- differences in density.
| MS 1a, b, c, 3b, c Students should be able to recall and apply this equation to changes where mass is conserved. |
Students should be able to recognise/draw simple diagrams to model the difference between solids, liquids and gases. | WS 1.2 |
Students should be able to explain the differences in density between the different states of matter in terms of the arrangement of atoms or molecules. | WS 1.2 |
Required practical activity 17 : use appropriate apparatus to make and record the measurements needed to determine the densities of regular and irregular solid objects and liquids. Volume should be determined from the dimensions of regularly shaped objects, and by a displacement technique for irregularly shaped objects. Dimensions to be measured using appropriate apparatus such as a ruler, micrometer or Vernier callipers.
AT skills covered by this practical activity: physics AT 1.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.3.1.2 Changes of state
Content | Key opportunities for skills development |
|---|
Students should be able to describe how, w hen substances change state (melt, freeze, boil, evaporate, condense or sublimate), mass is conserved. Changes of state are physical changes which differ from chemical changes because the material recovers its original properties if the change is reversed. | |
6.3.2 Internal energy and energy transfers
6.3.2.1 Internal energy
Content | Key opportunities for skills development |
|---|
Energy is stored inside a system by the particles (atoms and molecules) that make up the system. This is called internal energy. Internal energy is the total kinetic energy and potential energy of all the particles (atoms and molecules) that make up a system. Heating changes the energy stored within the system by increasing the energy of the particles that make up the system. This either raises the temperature of the system or produces a change of state. | |
6.3.2.2 Temperature changes in a system and specific heat capacity
Content | Key opportunities for skills development |
|---|
If the temperature of the system increases: The increase in temperature depends on the mass of the substance heated, the type of material and the energy input to the system. | |
The following equation applies: change in thermal energy, ∆ E , in joules, J mass, m , in kilograms, kg specific heat capacity, c , in joules per kilogram per degree Celsius, J/kg °C temperature change, ∆ θ , in degrees Celsius, °C. The specific heat capacity of a substance is the amount of energy required to raise the temperature of one kilogram of the substance by one degree Celsius. | MS 1a, 3b, 3c, 3d Students should be able to apply this equation, which is given on the Physics equation sheet , to calculate the energy change involved when the temperature of a material changes. This equation and specific heat capacity are also included in Energy changes in systems . |
6.3.2.3 Changes of state and specific latent heat
Content | Key opportunities for skills development |
|---|
If a change of state happens: The energy needed for a substance to change state is called latent heat. When a change of state occurs, the energy supplied changes the energy stored (internal energy) but not the temperature. The specific latent heat of a substance is the amount of energy required to change the state of one kilogram of the substance with no change in temperature. | |
energy, E , in joules, J mass, m , in kilograms, kg specific latent heat, L , in joules per kilogram, J/kg Specific latent heat of fusion – change of state from solid to liquid Specific latent heat of vaporisation – change of state from liquid to vapour | MS 1a, 3b, c, d Students should be able to apply this equation, which is given on the Physics equation sheet , to calculate the energy change involved in a change of state. MS 4a AT 5 Perform an experiment to measure the latent heat of fusion of water. |
Students should be able to interpret heating and cooling graphs that include changes of state. Students should be able to distinguish between specific heat capacity and specific latent heat. | WS 3.5 |
6.3.3 Particle model and pressure
6.3.3.1 Particle motion in gases
Content | Key opportunities for skills development |
|---|
The molecules of a gas are in constant random motion. The temperature of the gas is related to the average kinetic energy of the molecules. Changing the temperature of a gas, held at constant volume, changes the pressure exerted by the gas. | WS 1.2 |
Students should be able to: - explain how the motion of the molecules in a gas is related to both its temperature and its pressure
- explain qualitatively the relation between the temperature of a gas and its pressure at constant volume.
| WS 1.2 |
6.4 Atomic structure
Ionising radiation is hazardous but can be very useful. Although radioactivity was discovered over a century ago, it took many nuclear physicists several decades to understand the structure of atoms, nuclear forces and stability. Early researchers suffered from their exposure to ionising radiation. Rules for radiological protection were first introduced in the 1930s and subsequently improved. Today radioactive materials are widely used in medicine, industry, agriculture and electrical power generation.
6.4.1 Atoms and isotopes
6.4.1.1 The structure of an atom
Content | Key opportunities for skills development |
|---|
Atoms are very small, having a radius of about 1 × 10-10 metres. The basic structure of an atom is a positively charged nucleus composed of both protons and neutrons surrounded by negatively charged electrons. The radius of a nucleus is less than 1/10 000 of the radius of an atom. Most of the mass of an atom is concentrated in the nucleus. The electrons are arranged at different distances from the nucleus (different energy levels). The electron arrangements may change with the absorption of electromagnetic radiation (move further from the nucleus; a higher energy level) or by the emission of electromagnetic radiation (move closer to the nucleus; a lower energy level). | MS 1b WS 4.4 Students should be able to recognise expressions given in standard form. |
6.4.1.2 Mass number, atomic number and isotopes
Content | Key opportunities for skills development |
|---|
In an atom the number of electrons is equal to the number of protons in the nucleus. Atoms have no overall electrical charge. All atoms of a particular element have the same number of protons. The number of protons in an atom of an element is called its atomic number. The total number of protons and neutrons in an atom is called its mass number. Atoms can be represented as shown in this example: Atoms of the same element can have different numbers of neutrons; these atoms are called isotopes of that element. Atoms turn into positive ions if they lose one or more outer electron (s) . | |
Students should be able to relate differences between isotopes to differences in conventional representations of their identities, charges and masses. | WS 4.1 |
6.4.1.3 The development of the model of the atom (common content with chemistry)
Content | Key opportunities for skills development |
|---|
New experimental evidence may lead to a scientific model being changed or replaced. Before the discovery of the electron, atoms were thought to be tiny spheres that could not be divided. The discovery of the electron led to the plum pudding model of the atom. The plum pudding model suggested that the atom is a ball of positive charge with negative electrons embedded in it. The results from the alpha particle scattering experiment led to the conclusion that the mass of an atom was concentrated at the centre (nucleus) and that the nucleus was charged. This nuclear model replaced the plum pudding model. Niels Bohr adapted the nuclear model by suggesting that electrons orbit the nucleus at specific distances. The theoretical calculations of Bohr agreed with experimental observations. Later experiments led to the idea that the positive charge of any nucleus could be subdivided into a whole number of smaller particles, each particle having the same amount of positive charge. The name proton was given to these particles. The experimental work of James Chadwick provided the evidence to show the existence of neutrons within the nucleus. This was about 20 years after the nucleus became an accepted scientific idea. Students should be able to describe: | WS 1.1, 1.6 This historical context provides an opportunity for students to show an understanding of why and describe how scientific methods and theories develop over time. WS 1.2 |
- why the new evidence from the scattering experiment led to a change in the atomic model
| WS 1.1 |
- the difference between the plum pudding model of the atom and the nuclear model of the atom.
| WS 1.2 |
Details of experimental work supporting the Bohr model are not required. Details of Chadwick’s experimental work are not required. | |
6.4.2 Atoms and nuclear radiation
6.4.2.1 Radioactive decay and nuclear radiation
Content | Key opportunities for skills development |
|---|
Some atomic nuclei are unstable. The nucleus gives out radiation as it changes to become more stable. This is a random process called radioactive decay. Activity is the rate at which a source of unstable nuclei decay s . Activity is measured in becquerel (Bq) Count-rate is the number of decays recorded each second by a detector (eg Geiger-Muller tube). The nuclear radiation emitted may be: - an alpha particle (α) – this consists of two neutrons and two protons, it is the same as a helium nucleus
- a beta particle (β) – a high speed electron ejected from the nucleus as a neutron turns into a proton
- a gamma ray (γ) – electromagnetic radiation from the nucleus
- a neutron (n).
| |
Required knowledge of the properties of alpha particles, beta particles and gamma rays is limited to their penetration through materials, their range in air and ionising power. Students should be able to apply their knowledge to the uses of radiation and evaluate the best sources of radiation to use in a given situation. | WS 1.4 , 1.5 |
6.4.2.2 Nuclear equations
Content | Key opportunities for skills development |
|---|
Nuclear equations are used to represent radioactive decay. In a nuclear equation an alpha particle may be represented by the symbol: 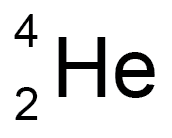 and a beta particle by the symbol: 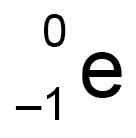 The emission of the different types of nuclear radiation may cause a change in the mass and /or the charge of the nucleus. For example: 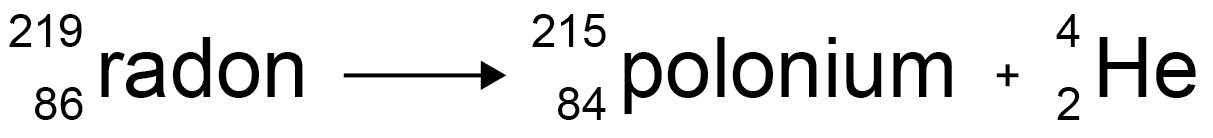 So alpha decay causes both the mass and charge of the nucleus to decrease. 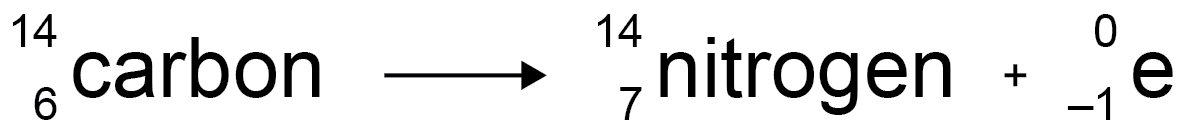 So beta decay does not cause the mass of the nucleus to change but does cause the charge of the nucleus to increase. Students are not required to recall these two examples. Students should be able to use the names and symbols of common nuclei and particles to write balanced equations that show single alpha (α) and beta (β) decay. This is limited to balancing the atomic numbers and mass numbers. The identification of daughter elements from such decays is not required. The emission of a gamma ray does not cause the mass or the charge of the nucleus to change. | WS 1.2, 4.1 MS 1b, c, 3c |
6.4.2.3 Half-lives and the random nature of radioactive decay
Content | Key opportunities for skills development |
|---|
Radioactive decay is random. The half-life of a radioactive isotope is the time it takes for the number of nuclei of the isotope in a sample to halve, or the time it takes for the count rate (or activity) from a sample containing the isotope to fall to half its initial level. | |
Students should be able to explain the concept of half-life and how it is related to the random nature of radioactive decay. | WS 1.2 |
Students should be able to determine the half-life of a radioactive isotope from given information. | MS 4a |
(HT only) Students should be able to calculate the net decline, expressed as a ratio, in a radioactive emission after a given number of half-lives. | (HT only) MS 1c, 3d |
6.4.2.4 Radioactive contamination
Content | Key opportunities for skills development |
|---|
Radioactive contamination is the unwanted presence of materials containing radioactive atoms on other materials. The hazard from contamination is due to the decay of the contaminating atoms. The type of radiation emitted affects the level of hazard. Irradiation is the process of exposing an object to nuclear radiation. The irradiated object does not become radioactive. | WS 1.5 |
Students should be able to compare the hazards associated with contamination and irradiation. | WS 1. 5 |
Suitable precautions must be taken to protect against any hazard that the radioactive source used in the process of irradiation may present. | WS 1.5 |
Students should understand that it is important for the findings of studies into the effects of radiation on humans to be published and shared with other scientists so that the findings can be checked by peer review. | WS 1.6 |
6.5 Forces
Engineers analyse forces when designing a great variety of machines and instruments, from road bridges and fairground rides to atomic force microscopes. Anything mechanical can be analysed in this way. Recent developments in artificial limbs use the analysis of forces to make movement possible.
6.5.1 Forces and their interactions
6.5.1.1 Scalar and vector quantities
Content | Key opportunities for skills development |
|---|
Scalar quantities have magnitude only. Vector quantities have magnitude and an associated direction. A vector quantity may be represented by an arrow. The length of the arrow represents the magnitude, and the direction of the arrow the direction of the vector quantity. | |
6.5.1.2 Contact and non-contact forces
Content | Key opportunities for skills development |
|---|
A force is a push or pull that acts on an object due to the interaction with another object. All forces between objects are either: - contact forces – the objects are physically touching
- non-contact forces – the objects are physically separated.
Examples of contact forces include friction, air resistance, tension and normal contact force. Examples of non-contact forces are gravitational force, electrostatic force and magnetic force. Force is a vector quantity. Students should be able to describe the interaction between pairs of objects which produce a force on each object. The forces to be represented as vectors. | |
6.5.1.3 Gravity
Content | Key opportunities for skills development |
|---|
Weight is the force acting on an object due to gravity. The force of gravity close to the Earth is due to the gravitational field around the Earth. The weight of an object depends on the gravitational field strength at the point where the object is. | |
The weight of an object can be calculated using the equation: weight, W , in newtons, N mass, m , in kilograms, kg gravitational field strength, g , in newtons per kilogram, N/kg (In any calculation the value of the gravitational field strength ( g ) will be given.) The weight of an object may be considered to act at a single point referred to as the object’s ‘centre of mass’. | MS 3b, c Students should be able to recall and apply this equation. |
The weight of an object and the mass of an object are directly proportional. Weight is measured using a calibrated spring-balance (a newtonmeter). | MS 3a Students should recognise and be able to use the symbol for proportionality, ∝ |
6.5.1.4 Resultant forces
Content | Key opportunities for skills development |
|---|
A number of forces acting on an object may be replaced by a single force that has the same effect as all the original forces acting together. This single force is called the resultant force. Students should be able to calculate the resultant of two forces that act in a straight line. (HT only) Students should be able to:- describe examples of the forces acting on an isolated object or system
| |
- use free body diagrams to describe qualitatively examples where several forces lead to a resultant force on an object, including balanced forces when the resultant force is zero.
(HT only) A single force can be resolved into two components acting at right angles to each other. The two component forces together have the same effect as the single force. | WS 1.2 |
(HT only) Students should be able to use vector diagrams to illustrate resolution of forces, equilibrium situations and determine the resultant of two forces, to include both magnitude and direction (scale drawings only). | MS 4a, 5a, b |
6.5.2 Work done and energy transfer
Content | Key opportunities for skills development |
|---|
When a force causes an object to move through a distance work is done on the object. So a force does work on an object when the force causes a displacement of the object. | |
The work done by a force on an object can be calculated using the equation: work done, W , in joules, J force, F , in newtons, N distance, s , in metres | MS 3b, c Students should be able to recall and apply this equation. |
One joule of work is done when a force of one newton causes a displacement of one metre. 1 joule = 1 newton - metre Students should be able to describe the energy transfer involved when work is done. | WS 4.5 |
Students should be able to convert between newton-metres and joules. Work done against the frictional forces acting on an object causes a rise in the temperature of the object. | MS 1c WS 4.5 |
6.5.3 Forces and elasticity
Content | Key opportunities for skills development |
|---|
Students should be able to: - give examples of the forces involved in stretching, bending or compressing an object
- explain why, to change the shape of an object (by stretching, bending or compressing), more than one force has to be applied – this is limited to stationary objects only
- describe the difference between elastic deformation and inelastic deformation caused by stretching forces.
The extension of an elastic object, such as a spring, is directly proportional to the force applied, provided that the limit of proportionality is not exceeded. | |
force, F , in newtons, N spring constant, k , in newtons per metre, N/m extension, e , in metres, m | MS 3b, c, 4a Students should be able to recall and apply this equation. |
This relationship also applies to the compression of an elastic object, where ‘ e ’ would be the compression of the object. A force that stretches (or compresses) a spring does work and elastic potential energy is stored in the spring. Provided the spring is not inelastically deformed, the work done on the spring and the elastic potential energy stored are equal. Students should be able to: - describe the difference between a linear and non-linear relationship between force and extension
- calculate a spring constant in linear cases
| MS 3b, c, 4a |
- interpret data from an investigation of the relationship between force and extension
| WS 3.5 |
| MS 3c Students should be able to apply this equation which is given on the Physics equation sheet . This equation is also given in Changes in energy . |
Students should be able to calculate relevant values of stored energy and energy transfers. | MS 3c |
Required practical activity 18: investigate the relationship between force and extension for a spring.
AT skills covered by this practical activity: physics AT 1 and 2.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.5.4 Forces and motion
6.5.4.1 Describing motion along a line
6.5.4.1.1 Distance and displacement
Content | Key opportunities for skills development |
|---|
Distance is how far an object moves. Distance does not involve direction. Distance is a scalar quantity. Displacement includes both the distance an object moves, measured in a straight line from the start point to the finish point and the direction of that straight line. Displacement is a vector quantity. Students should be able to express a displacement in terms of both the magnitude and direction. | MS 1, 3c Throughout this section (Forces and motion) , students should be able to use ratios and proportional reasoning to convert units and to compute rates. |
6.5.4.1.2 Speed
Content | Key opportunities for skills development |
|---|
Speed does not involve direction. Speed is a scalar quantity. The speed of a moving object is rarely constant. When people walk, run or travel in a car their speed is constantly changing. The speed at which a person can walk, run or cycle depends on many factors including: age, terrain, fitness and distance travelled. Typical values may be taken as: walking ̴ 1.5 m/s running ̴ 3 m/s cycling ̴ 6 m/s. Students should be able to recall typical values of speed for a person walking, running and cycling as well as the typical values of speed for different types of transportation systems. It is not only moving objects that have varying speed. The speed of sound and the speed of the wind also vary. A typical value for the speed of sound in air is 330 m/s. | |
Students should be able to make measurements of distance and time and then calculate speed s of objects . For an object moving at constant speed the distance travelled in a specific time can be calculated using the equation: | MS 1a, c, 2f |
distance, s , in metres, m speed, v , in metres per second, m/s time, t , in seconds, s | MS 3b, 3c Students should be able to recall and apply this equation. |
Students should be able to calculate average speed for non-uniform motion. | MS 3b, 3c |
6.5.4.1.3 Velocity
Content | Key opportunities for skills development |
|---|
The velocity of an object is its speed in a given direction. Velocity is a vector quantity. Students should be able to explain the vector–scalar distinction as it applies to displacement, distance, velocity and speed. (HT only) Students should be able to explain qualitatively, with examples, that motion in a circle involves constant speed but changing velocity . | |
6.5.4.1.4 The distance–time relationship
Content | Key opportunities for skills development |
|---|
If an object moves along a straight line, the distance travelled can be represented by a distance–time graph. The speed of an object can be calculated from the gradient of its distance–time graph. (HT only) If an object is accelerating, its speed at any particular time can be determined by drawing a tangent and measuring the gradient of the distance–time graph at that time. Students should be able to draw distance–time graphs from measurements and extract and interpret lines and slopes of distance–time graphs, translating information between graphical and numerical form. Students should be able to determine speed from a distance–time graph. | MS 4a, b, c, d, f |
6.5.4.1.5 Acceleration
Content | Key opportunities for skills development |
|---|
The average acceleration of an object can be calculated using the equation: | |
acceleration, a , in metres per second squared, m/s2 change in velocity, ∆ v , in metres per second, m/s time, t , in seconds, s An object that slows down is decelerating . Students should be able to estimate the magnitude of everyday accelerations. | MS 1d, 3b, 3c Students should be able to recall and apply this equation. |
The acceleration of an object can be calculated from the gradient of a velocity–time graph. (HT only) The distance travelled by an object (or displacement of an object) can be calculated from the area under a velocity–time graph. Students should be able to: | MS 4a, b, c, d, f |
- draw velocity–time graphs from measurements and interpret lines and slopes to determine acceleration
- (HT only) interpret enclosed areas in velocity–time graphs to determine distance travelled (or displacement)
| WS 3.3 |
- (HT only) measure, when appropriate, the area under a velocity–time graph by counting squares.
| WS 3.3 |
The following equation applies to uniform acceleration : final velocity, v , in metres per second, m/s initial velocity, u , in metres per second, m/s acceleration, a , in metres per second squared, m/s2 distance, s , in metres, m Near the Earth’s surface any object falling freely under gravity has an acceleration of about 9.8 m/s2 . | MS 3b, 3c Students should be able to apply this equation which is given on the Physics equation sheet . |
An object falling through a fluid initially accelerates due to the force of gravity. Eventually the resultant force will be zero and the object will move at its terminal velocity. | |
6.5.4.2 Forces, accelerations and Newton's Laws of motion
6.5.4.2.1 Newton's First Law
Content | Key opportunities for skills development |
|---|
Newton’s First Law: If the resultant force acting on an object is zero and: - the object is stationary, the object remains stationary
- the object is moving, the object continues to move at the same speed and in the same direction. So the object continues to move at the same velocity.
So, when a vehicle travels at a steady speed the resistive forces balance the driving force. So, the velocity (speed and/or direction) of an object will only change if a resultant force is acting on the object. Students should be able to apply Newton’s First Law to explain the motion of objects moving with a uniform velocity and objects where the speed and/or direction changes. (HT only) The tendency of objects to continue in their state of rest or of uniform motion is called inertia. | |
6.5.4.2.2 Newton's Second Law
Content | Key opportunities for skills development |
|---|
Newton’s Second Law: | |
The acceleration of an object is proportional to the resultant force acting on the object, and inversely proportional to the mass of the object. As an equation: | MS 3a Students should recognise and be able to use the symbol for proportionality, ∝ |
force, F , in newtons, N mass, m , in kilograms, kg acceleration, a , in metres per second squared, m/s2 | MS 3b, c WS 4.2 Students should be able to recall and apply this equation. |
(HT only) Students should be able to explain that: - inertial mass is a measure of how difficult it is to change the velocity of an object
- inertial mass is defined as the ratio of force over acceleration .
| MS 3a |
Students should be able to estimate the speed, accelerations and forces involved in large accelerations for everyday road transport. Students should recognise and be able to use the symbol that indicates an approximate value or approximate answer, ̴ | MS 1d |
Required practical activity 19 : investigate the effect of varying the force on the acceleration of an object of constant mass, and the effect of varying the mass of an object on the acceleration produced by a constant force.
AT skills covered by this practical activity: physics AT 1, 2 and 3.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.5.4.2.3 Newton's Third Law
Content | Key opportunities for skills development |
|---|
Newton’s Third Law: | |
Whenever two objects interact, the forces they exert on each other are equal and opposite. Students should be able to apply Newton’s Third Law to examples of equilibrium situations. | WS 1.2 |
6.5.4.3 Forces and braking
6.5.4.3.1 Stopping distance
Content | Key opportunities for skills development |
|---|
The stopping distance of a vehicle is the sum of the distance the vehicle travels during the driver’s reaction time (thinking distance) and the distance it travels under the braking force (braking distance). For a given braking force the greater the speed of the vehicle, the greater the stopping distance. | |
6.5.4.3.2 Reaction time
Content | Key opportunities for skills development |
|---|
Reaction times vary from person to person. Typical values range from 0. 2 s to 0.9 s. A driver’s reaction time can be affected by tiredness, drugs and alcohol. Distractions may also affect a driver’s ability to react. Students should be able to: - explain methods used to measure human reaction times and recall typical results
| |
- interpret and evaluate measurements from simple methods to measure the different reaction times of students
| WS 3.5, 3.7 |
- evaluate the effect of various factors on thinking distance based on given data.
| WS 1.5, 2.2 MS 1a, c AT 1 Measure the effect of distractions on reaction time. |
6.5.4.3.3 Factors affecting braking distance 1
Content | Key opportunities for skills development |
|---|
The braking distance of a vehicle can be affected by adverse road and weather conditions and poor condition of the vehicle. Adverse road conditions include wet or icy conditions. Poor condition of the vehicle is limited to the vehicle's brakes or tyres. Students should be able to: - explain the factors which affect the distance required for road transport vehicles to come to rest in emergencies, and the implications for safety
| |
- estimate how the distance required for road vehicles to stop in an emergency varies over a range of typical speeds.
| MS 1c, 1d, 2c, 2d, 2f, 2h, 3b, 3c |
6.5.4.3.4 Factors affecting braking distance 2
Content | Key opportunities for skills development |
|---|
When a force is applied to the brakes of a vehicle, work done by the friction force between the brakes and the wheel reduces the kinetic energy of the vehicle and the temperature of the brakes increases. The greater the speed of a vehicle the greater the braking force needed to stop the vehicle in a certain distance. The greater the braking force the greater the deceleration of the vehicle. Large decelerations may lead to brakes overheating and/or loss of control. Students should be able to: | |
- explain the dangers caused by large decelerations
| WS 1.5 |
- (HT only) estimate the forces involved in the deceleration of road vehicles in typical situations on a public road.
| (HT only) MS 1d |
6.5.5 Momentum (HT only)
6.5.5.1 Momentum is a property of moving objects
Content | Key opportunities for skills development |
|---|
Momentum is defined by the equation: momentum, p , in kilograms metre per second, kg m/s mass, m , in kilograms, kg velocity, v , in metres per second, m/s | WS 1.2 MS 3b, c Students should be able to recall and apply this equation. |
6.5.5.2 Conservation of momentum
Content | Key opportunities for skills development |
|---|
In a closed system, the total momentum before an event is equal to the total momentum after the event. This is called conservation of momentum. Students should be able to use the concept of momentum as a model to describe and explain examples of momentum in a n event, such as a collision. | AT 1, 2, 3 Investigate collisions between laboratory trollies using light gates, data loggers or ticker timers to measure and record data. |
6.6 Waves
Wave behaviour is common in both natural and man-made systems. Waves carry energy from one place to another and can also carry information. Designing comfortable and safe structures such as bridges, houses and music performance halls requires an understanding of mechanical waves. Modern technologies such as imaging and communication systems show how we can make the most of electromagnetic waves.
6.6.1 Waves in air, fluids and solids
6.6.1.1 Transverse and longitudinal waves
Content | Key opportunities for skills development |
|---|
Waves may be either transverse or longitudinal. The ripples on a water surface are an example of a transverse wave. Longitudinal waves show areas of compression and rarefaction. Sound waves travelling through air are longitudinal. | |
Students should be able to describe the difference between longitudinal and transverse waves. | WS 1.2 |
Students should be able to describe evidence that, for both ripples on a water surface and sound waves in air, it is the wave and not the water or air itself that travels. | WS 1.2, 2.2 |
6.6.1.2 Properties of waves
Content | Key opportunities for skills development |
|---|
Students should be able to describe wave motion in terms of their amplitude, wavelength, frequency and period. The amplitude of a wave is the maximum displacement of a point on a wave away from its undisturbed position. The wavelength of a wave is the distance from a point on one wave to the equivalent point on the adjacent wave. The frequency of a wave is the number of waves passing a point each second. | MS 1c, 3b, c |
period, T , in seconds, s frequency, f , in hertz, Hz The wave speed is the speed at which the energy is transferred (or the wave moves) through the medium. All waves obey the wave equation: | MS 1c, 3b, c Students should be able to apply this equation which is given on the Physics equation sheet . |
wave speed, v , in metres per second, m/s frequency, f , in hertz, Hz wavelength, λ , in metres, m Students should be able to: - identify amplitude and wavelength from given diagrams
| MS 1c, 3b, 3c Students should be able to recall and apply this equation. |
- describe a method to measure the speed of sound waves in air
| AT 1 WS 2.3, 2.4, 2.6, 2.7, 3.1, 3.5 |
- describe a method to measure the speed of ripples on a water surface.
| AT 1, AT 4 WS 2.3, 2.4, 2.6, 2.7, 3.1, 3.5 |
Required practical activity 20: make observations to identify the suitability of apparatus to measure the frequency, wavelength and speed of waves in a ripple tank and waves in a solid and take appropriate measurements.
AT skills covered by this practical activity: physics AT 4.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.6.2 Electromagnetic waves
6.6.2.1 Types of electromagnetic waves
Content | Key opportunities for skills development |
|---|
Electromagnetic waves are transverse waves that transfer energy from the source of the waves to an absorber. Electromagnetic waves form a continuous spectrum and all types of electromagnetic wave travel at the same velocity through a vacuum (space) or air. The waves that form the electromagnetic spectrum are grouped in terms of their wavelength and their frequency. Going from long to short wavelength (or from low to high frequency) the groups are: radio, microwave, infrared, visible light (red to violet), ultraviolet, X-rays and gamma rays. 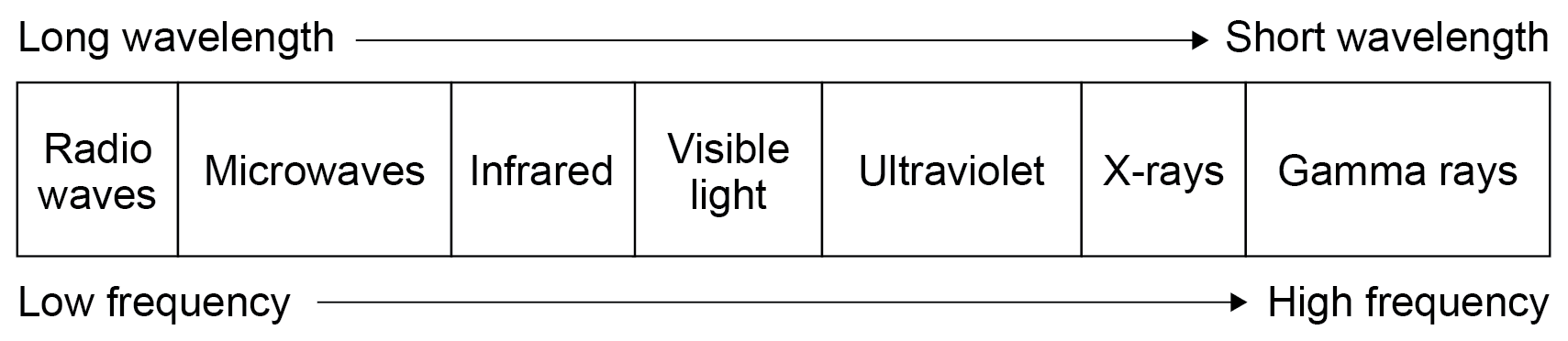 Our eyes only detect visible light and so detect a limited range of electromagnetic waves. Students should be able to give examples that illustrate the transfer of energy by electromagnetic waves. | |
6.6.2.2 Properties of electromagnetic waves 1
Content | Key opportunities for skills development |
|---|
(HT only) Different substances may absorb, transmit, refract or reflect electromagnetic waves in ways that vary with wavelength . (HT only) Some effects, for example refraction, are due to the difference in velocity of the waves in different substances. Students should be able to construct ray diagrams to illustrate the refraction of a wave at the boundary between two different media. | |
| (HT only) Students should be able to use wave front diagrams to explain refraction in terms of the change of speed that happens when a wave travels from one medium to a different medium. | WS 1.2 |
Required practical activity 21: investigate how the amount of infrared radiation absorbed or radiated by a surface depends on the nature of that surface.
AT skills covered by this practical activity: physics AT 1 and 4.
This practical activity also provides opportunities to develop WS and MS. Details of all skills are given in Key opportunities for skills development .
6.6.2.3 Properties of electromagnetic waves 2
Content | Key opportunities for skills development |
|---|
(HT only) Radio waves can be produced by oscillations in electrical circuits. (HT only) When radio waves are absorbed they may create an alternating current with the same frequency as the radio wave itself, so radio waves can themselves induce oscillations in an electrical circuit. Changes in atoms and the nuclei of atoms can result in electromagnetic waves being generated or absorbed over a wide frequency range. Gamma rays originate from changes in the nucleus of an atom. | |
Ultraviolet waves, X-rays and gamma rays can have hazardous effects on human body tissue. The effects depend on the type of radiation and the size of the dose. Radiation dose (in sieverts) is a measure of the risk of harm resulting from an exposure of the body to the radiation. | WS 1.5 |
1000 millisieverts (mSv) = 1 sievert (Sv) Students will not be required to recall the unit of radiation dose. Students should be able to draw conclusions from given data about the risks and consequences of exposure to radiation. Ultraviolet waves can cause skin to age prematurely and increase the risk of skin cancer. X-rays and gamma rays are ionising radiation that can cause the mutation of genes and cancer. | WS 1.5 |
6.6.2.4 Uses and applications of electromagnetic waves
Content | Key opportunities for skills development |
|---|
Electromagnetic waves have many practical applications. For example: - radio waves – television and radio
- microwaves – satellite communications, cooking food
- infrared – electrical heaters, cooking food, infrared cameras
- visible light – fibre optic communications
- ultraviolet – energy efficient lamps, sun tanning
- X-rays and gamma rays – medical imaging and treatments.
| |
(HT only) Students should be able to give brief explanations why each type of electromagnetic wave is suitable for the practical application. | (HT only) WS 1.4 |
6.7 Magnetism and electromagnetism
Electromagnetic effects are used in a wide variety of devices. Engineers make use of the fact that a magnet moving in a coil can produce electric current and also that when current flows around a magnet it can produce movement. It means that systems that involve control or communications can take full advantage of this.
6.7.1 Permanent and induced magnetism, magnetic forces and fields
6.7.1.1 Poles of a magnet
Content | Key opportunities for skills development |
|---|
The poles of a magnet are the places where the magnetic forces are strongest. When two magnets are brought close together they exert a force on each other. Two like poles repel each other. Two unlike poles attract each other. Attraction and repulsion between two magnetic poles are examples of non-contact force. A permanent magnet produces its own magnetic field. An induced magnet is a material that becomes a magnet when it is placed in a magnetic field. Induced magnetism always causes a force of attraction. When removed from the magnetic field an induced magnet loses most/all of its magnetism quickly. Students should be able to describe: - the attraction and repulsion between unlike and like poles for permanent magnets
- the difference between permanent and induced magnets.
| |
6.7.1.2 Magnetic fields
Content | Key opportunities for skills development |
|---|
The region around a magnet where a force acts on another magnet or on a magnetic material (iron, steel, cobalt and nickel) is called the magnetic field. The force between a magnet and a magnetic material is always one of attraction. The strength of the magnetic field depends on the distance from the magnet. The field is strongest at the poles of the magnet. The direction of the magnetic field at any point is given by the direction of the force that would act on another north pole placed at that point. The direction of a magnetic field line is from the north (seeking) pole of a magnet to the south(seeking) pole of the magnet. A magnetic compass contains a small bar magnet. The Earth has a magnetic field. The compass needle points in the direction of the Earth’s magnetic field. Students should be able to: - describe how to plot the magnetic field pattern of a magnet using a compass
- draw the magnetic field pattern of a bar magnet showing how strength and direction change from one point to another
- explain how the behaviour of a magnetic compass is related to evidence that the core of the Earth must be magnetic.
| WS 2.2 |
6.7.2 The motor effect
6.7.2.1 Electromagnetism
Content | Key opportunities for skills development |
|---|
When a current flows through a conducting wire a magnetic field is produced around the wire. The strength of the magnetic field depends on the current through the wire and the distance from the wire. Shaping a wire to form a solenoid increases the strength of the magnetic field created by a current through the wire. The magnetic field inside a solenoid is strong and uniform. The magnetic field around a solenoid has a similar shape to that of a bar magnet. Adding an iron core increases the strength of the magnetic field of a solenoid . An electromagnet is a solenoid with an iron core. Students should be able to: | |
- describe how the magnetic effect of a current can be demonstrated
- draw the magnetic field pattern for a straight wire carrying a current and for a solenoid (showing the direction of the field)
- explain how a solenoid arrangement can increase the magnetic effect of the current.
| WS 2.2 |
6.7.2.2 Fleming's left-hand rule (HT only)
Content | Key opportunities for skills development |
|---|
When a conductor carrying a current is placed in a magnetic field the magnet producing the field and the conductor exert a force on each other. This is called the motor effect. Students should be able to show that Fleming's left-hand rule represents the relative orientation of the force, the current in the conductor and the magnetic field. Students should be able to recall the factors that affect the size of the force on the conductor. | |
For a conductor at right angles to a magnetic field and carrying a current: force, F , in newtons, N magnetic flux density, B , in tesla, T current, I , in amperes, A (amp is acceptable for ampere) length, l , in metres, m | MS 3b, c Students should be able to apply this equation which is given on the physics equation sheet . |
6.7.2.3 Electric motors (HT only)
Content | Key opportunities for skills development |
|---|
A coil of wire carrying a current in a magnetic field tends to rotate. This is the basis of an electric motor. Students should be able to explain how t he force on a conductor in a magnetic field causes the rotation of the coil in an electric motor. | |
6.8 Key ideas
The complex and diverse phenomena of the natural and man-made world can be described in terms of a small number of key ideas in physics.
These key ideas are of universal application, and we have embedded them throughout the subject content. They underpin many aspects of the science assessment and will therefore be assessed across all papers.
Key ideas in physics:
- the use of models, as in the particle model of matter or the wave models of light and of sound
- the concept of cause and effect in explaining such links as those between force and acceleration, or between changes in atomic nuclei and radioactive emissions
- the phenomena of ‘action at a distance’ and the related concept of the field as the key to analysing electrical, magnetic and gravitational effects
- that differences, for example between pressures or temperatures or electrical potentials, are the drivers of change
- that proportionality, for example between weight and mass of an object or between force and extension in a spring, is an important aspect of many models in science
- that physical laws and models are expressed in mathematical form.